Class X - Maths Basic Sample Paper - 1

Kindly share you feedback about the website – Click here

General Instructions:

1. This Question Paper has 5 Sections A, B, C, D, and E.

2. Section A has 20 Multiple Choice Questions (MCQs) carrying 1 mark each.

3. Section B has 5 Short Answer-I (SA-I) type questions carrying 2 marks each.

4. Section C has 6 Short Answer-II (SA-II) type questions carrying 3 marks each.

5. Section D has 4 Long Answer (LA) type questions carrying 5 marks each.

6. Section E has 3 sourced based/Case Based/passage based/integrated units of assessment (4 marks each) with sub-parts of the values of 1, 1 and 2 marks each respectively.

7. All Questions are compulsory. However, an internal choice in 2 Qs of 2 marks, 2 Qs of 3 marks and 2 Questions of 5 marks has been provided. An internal choice has been provided in the 2 marks questions of Section E.

8. Draw neat figures wherever required. Take π =22/7 wherever required if not stated.

SECTION A

1. If two positive integers a and b are written as a = x3y 2 and b = xy3 ; where x, y are prime numbers, then HCF (a, b) is:

a) xy b) xy² c) x³y ³ d) x²y ²

View Answer

Ans. b) xy²

2. The LCM of smallest two-digit composite number and smallest composite number is:

a) 12 b) 4 c) 20 d) 44

View Answer

Ans. c) 20

3. If x = 3 is one of the roots of the quadratic equation x² – 2kx – 6 = 0, then the value of k is

a) – 1/2 b) 1/2 c) 3 d) 2

View Answer

Ans. b) 1/2

4. The pair of equations y = 0 and y = -7 has:

a) one solution b) two solutions c) infinitely many solutions d) no solution

View Answer

Ans. d) no solution

5. Value(s) of k for which the quadratic equation 2x² – kx + k = 0 has equal roots is :

a) 0 only b) 4 c) 8 only d) 0,8

View Answer

Ans. d) 0,8

6. The distance of the point (3, 5) from x-axis is k units, then k equals:

a) 3 b) 4 c) 5 d) 8

View Answer

Ans. c) 5

7. If in ∆ ABC and ∆ PQR, AB/QR = BC/PR = CA/PQ then:

a) ∆PQR ~∆CAB b) ∆PQR ~∆ABC c) ∆CBA ~∆PQR d) ∆BCA ~∆PQR

View Answer

Ans. a) ∆PQR ~∆CAB

8. Which of the following is NOT a similarity criterion of traingles?

a) AA b) SAS c) AAA d) RHS

View Answer

Ans. d) RHS

9. In figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to

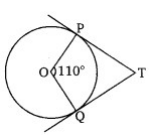
(a) 60° (b) 70° (c) 80° (d) 90°

View Answer

Ans. (b) 70°

10. If cos A = 4/5 then tan A is:

a) 3/5 b) ¾ c) 4/3 d) 1/8

View Answer

Ans. b) ¾

11. If the height of the tower is equal to the length of its shadow, then the angle of elevation of the sun is _____

a) 30° b) 45° c) 60° d) 90°

View Answer

Ans. b) 45°

12. (1 – cos² A) is equal to

a) sin² A b) tan²A c) 1 – sin²A d) sec²A

View Answer

Ans. a) sin² A

13. The radius of a circle is same as the side of a square. Their perimeters are in the ratio

a) 1 : 1 b) 2 : 𝜋 c) 𝜋 : 2 d) √𝜋 : 2

View Answer

Ans. c) 𝜋 : 2

14. The area of the circle is 154cm². The radius of the circle is

a) 7cm b) 14cm c) 3.5cm d) 17.5cm

View Answer

Ans. a) 7cm

15. When a dice is thrown once, the probability of getting an even number less than 4 is

a) 1/4 b) 0 c) 1/2 d) 1/6

View Answer

Ans. d) 1/6

16. For the following distribution:

The lower limit of modal class is:

a) 15 b) 20 c) 10 d) 5

View Answer

Ans. a) 15

17. A rectangular sheet of paper 40cm x 22cm, is rolled to form a hollow cylinder of height 40cm. The radius of the cylinder (in cm) is :

a) 3.5 b) 7 c) 80/7 d) 5

View Answer

Ans. a) 3.5

18. Consider the following frequency distribution:

The median class is:

a) 6-12 b) 12-18 c) 18-24 d) 24-30

View Answer

Ans. b) 12-18

19. Assertion (A): The point (0, 4) lies on y-axis.

Reason(R): The x-coordinate of a point on y-axis is zero

(a) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A).

(b) Both assertion (A) and reason (R) are true but reason (R) is not the correct explanation of assertion (A).

(d) Assertions (A) is false but reason (R) is true.

View Answer

Ans. (a) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A).

20. Assertion (A): The HCF of two numbers is 5 and their product is 150. Then their LCM is 40.

Reason(R): For any two positive integers a and b, HCF (a, b) x LCM (a, b) = a x b.

(a) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A).

(b) Both assertion (A) and reason (R) are true but reason (R) is not the correct explanation of assertion (A).

(d) Assertions (A) is false but reason (R) is true.

View Answer

Ans. (d) Assertions (A) is false but reason (R) is true.

SECTION B

21. Find whether the following pair of linear equations is consistent or inconsistent:

3x + 2y = 8

6x – 4y = 9

View Answer

Ans.

3x + 2y = 8

6x – 4y = 9

a₁ = 3 b₁ = 2 c₁ = 8

a₂ = 6 b₂ = 4 c₂ = 9

a₁/a₂ = 3/6 = 1/2 b₁/b₂ = 2/-4 = -1/2 c₁/c₂ = 8/9

a₁/a₂ ≠ b₁/b₂

The given pair of linear equations are consistent.

22. In the given figure, if ABCD is a trapezium in which AB║CD ║ EF, then prove that AE/ED = BF/FC

View Answer

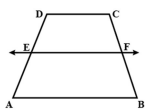
Ans.

Given:-AB II CD II EF

To prove:- AE/ED = BF/FC

Construction:- Join BD to intersect EF at G.

Proof:- in ∆ ABD

EG II AB (EF II AB)

AE/ED = BG/GD (by BPT)___________(1)

In ∆𝐷𝐵𝐶

GF II CD (EF II CD)

BF/FC = BG/GD (by BPT) ___________(2)

𝑓𝑟𝑜𝑚 (1) & (2)

AE/ED = BF/FC

In figure, if AD = 6cm, DB = 9cm, AE = 8cm and EC = 12cm and ∠ADE = 48˚. Find ∠ABC.

View Answer

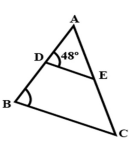
Ans.

Given AD=6cm, DB=9cm

AE=8cm, EC=12cm, ∠ADE=48

To find:- ∠ABC=?

Proof: In ∆𝐴𝐵𝐶

AD/DB = 6/9 = 2/3 ……(1)

AE/EC = 8/12 = 2/3 ……..(2)

From (1) & (2)

AD/DB = AE/EC

DE II BC (Converse of BPT)

∠ADE=∠ABC (Corresponding angles)

⇒ ∠ABC=48˚

23. The length of a tangent from a point A at distance 5cm from the centre of the circle is 4cm. Find the radius of the circle.

View Answer

Ans.

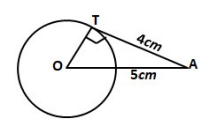
In ∆ OTA, ∠OTA = 90˚

By Pythagoras theorem

OA² = OT² + AT²

(5)² = OT² + (4)²

25-16= OT²

9 = OT²

OT= 3cm

radius of circle = 3cm.

24. Evaluate: sin² 60˚ + 2tan 45˚ – cos² 30˚.

View Answer

Ans. Sin² 60˚ + 2 tan 45˚ – cos² 30˚

= (√3/2)² + 2(1) – (√3/2)²

= ¾ + 2 – ¾

= 2

25. Find the diameter of a circle whose area is equal to the sum of the areas of two circles of radii 40cm and 9cm.

View Answer

Ans. Area of the circle= sum of areas of 2 circles

𝜋𝑅² = 𝜋(40)² + 𝜋(9)²

𝜋𝑅² = 𝜋 x (40² + 9²)

𝑅²= 1600 + 81

𝑅² = 1681

𝑅 = 41 𝑐𝑚.

𝐷𝑖𝑎𝑚𝑒𝑡𝑒𝑟 𝑜𝑓 𝑔𝑖𝑣𝑒𝑛 𝑐𝑖𝑟𝑐𝑙𝑒 = 41 × 2 = 82𝑐𝑚

A chord of a circle of radius 10cm subtends a right angle at the centre. Find the area of minor segment. (Use 𝜋 = 3.14)

View Answer

Ans. radius of circle = 10cm, 𝜃 = 90˚

Area of minor segment = 𝜃/360˚ 𝜋r² – Area of Δ

= 𝜃/360˚ x 𝜋 r² – 1/2 x b x h

= 90/360 x 3.13 x 10 x 10 – 1/2x 10 x 10

= 314/4 – 50

= 78.5 – 50

= 28.5 cm²

Area of minor segment = 28.5 cm²

SECTION C

26. Prove that √3 is an irrational number.

View Answer

Ans. Let us assume that √3 be a rational number

√3 = a/b where a and b are co-prime.

squaring both the sides

(√3)² = (a/b)²

3=a²/b²

⇒ 𝑎 ²=3𝑏 b²

𝑎 ² is divisible by 3 so a is also divisible by 3_________(1)

𝑙𝑒𝑡 a=3c for any integer c.

(3𝑐) ²=3b²

9𝑐²= 3𝑏²

𝑏²= 3𝑐²

𝑠𝑖𝑛𝑐𝑒 𝑏² is divisible by 3 so, b is also divisible by 3 _____(2)

From (1) & (2) we can say that 3 in a factor of a and b

which is contradicting the fact that a and b are co- prime.

Thus, our assumption that √3 is a rational number is wrong.

Hence, √3 is an irrational number.

27. Find the zeroes of the quadratic polynomial 4s² – 4s + 1 and verify the relationship between the zeroes and the coefficients.

View Answer

Ans. P(S)= 4S² -4S+1

4S² -2S-2S+1=0

2S(2S-1) -1(2S-1) = 0

(2S-1) (2S-1) = 0

S = ½ S = ½

a = 4 b = – 4 c = 1 ∝ = ½ 𝛽 = ½

∝ +𝛽 = -b/a, ∝ 𝛽 = c/a,

½ + ½ = -4/4, (1/2)(1/2) = ¼

(1 + 1)/2 = +4/4, ¼ = ¼

2/2 = 1

1 = 1

28. The coach of a cricket team buys 4 bats and 1 ball for Rs. 2050. Later, she buys 3 bats and 2 balls for 1600. Find the cost of each bat and each ball.

View Answer

Ans. Let cost of one bat be Rs 𝑥

Let cost of one ball be Rs 𝑦

ATQ

4𝑥 + 1𝑦 = 2050___________(1)

3𝑥 + 2𝑦 = 1600___________(2)

𝑓𝑟𝑜𝑚 (1)4𝑥 + 1𝑦 = 2050 𝑦 = 2050 − 4𝑥

𝑦 = 2050 – 4x

𝑆𝑢𝑏𝑠𝑡𝑖𝑡𝑒 𝑣𝑎𝑙𝑢𝑒 𝑜𝑓 𝑦 𝑖𝑛 (2)

3𝑥 + 2(2050 − 4𝑥) = 1600

3x + 4100 – 8x =1600

-5x = −2500

𝑥 = 500

𝑆𝑢𝑏𝑠𝑡𝑖𝑡𝑢𝑟𝑒 𝑣𝑎𝑙𝑢𝑒 𝑜𝑓 𝑥 𝑖𝑛 (1)

4𝑥 + 1𝑦 = 2050

4(500) + 𝑦 = 2050

2000 + 𝑦 = 2050

𝑦 = 50

Hence

Cost of one bat = Rs. 500

Cost of one ball = Rs. 50

A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Saritha paid ₹27 for a book kept for seven days, while Susy paid ₹21 for the book she kept for five days. Find the fixed charge and the charge for each extra day.

View Answer

Ans. Let the fixed charge for first 3 days= Rs. 𝑥

And additional charge after 3 days= Rs. 𝑦

ATQ

𝑥 + 4𝑦 = 27—————(1)

𝑥 + 2𝑦 = 21 ————–(2)

Subtract eqn (2) from (1)

2𝑦 = 6

𝑦 = 3

Substitute value of 𝑦 in (2)

𝑥 + 2(3) = 21

𝑥 = 21 − 6

𝑥 = 15

Fixed charge= Rs. 15

Additional charge per day = Rs. 3

29. A circle touches all the four sides of quadrilateral ABCD. Prove that AB + CD = AD + BC.

View Answer

Ans. Given circle touching sides of ABCD at P, Q, R and S

To prove- AB+CD=AD+BC

Proof-

AP=AS——-(1) tangents from an external point

PB=BQ——-(2) to a circle are equal in length

DR=DS——-(3)

CR=CQ——-(4)

Adding eqn (1), (2), (3) & (4)

AP+BP+DR+CR=AS+DS+BQ+CQ

AB+DC=AD+BC

30. Prove that

(cosec 𝜃 – cot 𝜃) ² = (1 – cos 𝜃)/ (1 + cos 𝜃)

View Answer

Ans.

Prove that sec A (1 – sin A) (sec A + tan A) = 1.

View Answer

Ans.

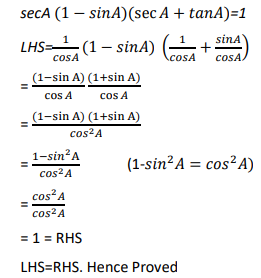
31. A bag contains 6 red, 4 black and some white balls.

(i) Find the number of white balls in the bag if the probability of drawing a white ball is 1/3.

View Answer

Ans. Red balls= 6, Black balls = 4, White balls = x

P(white ball) = x/(10 + x) = 1/3

⇒ 3x = 10 + x ⇒ x= 5 white balls

(ii) How many red balls should be removed from the bag for the probability of drawing a white ball to be 1/2?

View Answer

Ans. Let y red balls be removed, black balls = 4, white balls = 5

P(white balls)= 5/[(6 – y) + 4 + 5] = ½

5/(15 – y) = ½ ⇒ 10 = 15 − 𝑦 ⇒ 𝑦 = 5

So 5 balls should be removed

SECTION D

32. A train travels 360km at a uniform speed. If the speed had been 5km/h more, it would have taken 1 hour less for the same journey. Find the speed of the train.

View Answer

Ans. Let the speed of train be 𝑥 𝑘𝑚/ℎ𝑟

distance= 360 km

Speed = Distance/Time

Time = 360/x

New speed = (𝑥 + 5) 𝑘𝑚/ℎ𝑟

Time = D/S

𝑥 + 5 = 360/[(360/x) – 1]

(𝑥 + 5) (360/x – 1) = 360

(𝑥 + 5)(360 − 𝑥) = 360𝑥

−𝑥 ² − 5𝑥 + 1800 = 0

𝑥 ² + 5𝑥 − 1800 = 0

𝑥² + 45𝑥 − 40𝑥 − 1800 = 0

𝑥(𝑥 + 45) − 40(𝑥 + 45) = 0

(𝑥 + 45) (𝑥 − 40) = 0

𝑥 + 45 = 0, 𝑥 − 40 = 0

𝑥 = −45, 𝑥 = 40

Speed cannot be negative

Speed of train =40km/hr

A motor boat whose speed is 18km/h in still water takes 1 hour more to go 24km upstream than to return downstream to the same spot. Find the speed of the stream.

View Answer

Ans. Let the speed of the stream=𝑥𝑘𝑚/ℎ𝑟

Speed of boat= 18 𝑘𝑚/ℎ𝑟

Upstream speed= (18 − 𝑥)𝑘𝑚/ℎ𝑟

Downstream speed=(18 + 𝑥)𝑘𝑚/ℎ𝑟

Time taken (upstream)= 24/(18 – x)

Time taken (downstream)= 24/(18 + x)

ATQ

24/(18 – x) = 24/(18 + x) + 1

24/(18 – x) – 24/(18 + x) = 1

24(18 + 𝑥) − 24(18 − 𝑥) = (18 − 𝑥)(18 + 𝑥)

24(18 + 𝑥 − 18 + 𝑥) = (18)² − 𝑥 ²

24(2𝑥) = 324 − 𝑥 ²

48𝑥 − 324 + 𝑥 ² = 0

𝑥 ² + 48𝑥 − 324 = 0

𝑥 ² − 6𝑥 + 54𝑥 − 324 = 0

𝑥(𝑥 − 6) + 54(𝑥 − 6) = 0

(𝑥 − 6) (𝑥 + 54) = 0

𝑥 − 6 = 0, 𝑥 + 54 = 0

𝑥 = 6, 𝑥 = −54

Speed cannot be negative

Speed of stream=6𝑘𝑚/ℎr

33. Prove that If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.

View Answer

Ans. Given ∆ 𝐴𝐵𝐶, DE || BC

To prove AD/DB = AE/EC

Construction: join BE and CD

Draw DM ⏊ AC and EN ⏊ AB

Proof: 𝐴𝑟𝑒𝑎 𝑜𝑓 ∆𝐴𝐷𝐸 = ½ x b x h

= ½ x AD x EN———————–(1)

Area (∆𝐷𝐵𝐸) = ½ x DB x EN——–(2)

Divide eqn (1) by (2)

ar ∆ADE/ar ∆DBE = ½ x AD x EN/1/2 x DB x EN = AD/DB ———–(3)

area ∆𝐴𝐷𝐸 = ½ x AE x DM ——-(4)

area ∆𝐷𝐸𝐶 = ½ x EC x DM ——-(5)

Divide eqn (4) by (5)

ar ∆ADE/ar ∆DEC = ½ x AE x DM/ ½ x EC x DM = AE/EC ———–(6)

∆𝐵𝐷𝐸 and ∆𝐷𝐸𝐶 are on the same base DE and between same parallel lines BC and DE

∴ 𝑎𝑟𝑒𝑎 (∆𝐷𝐵𝐸) = 𝑎𝑟 ( 𝐷𝐸𝐶)

In ΔPQR, S and T are points on PQ and PR respectively. PS/SQ = PT/TR and ∠PST = ∠PRQ. Prove that PQR is an isosceles triangle.

Hence

ar (ΔADE)/ar (ΔDBE) = ar(ΔADE)/ar(ΔDEC) [LHS of (3) = RHS of (6)]

AD/DB = AE/EC [RHS of (3) = RHS of (6)

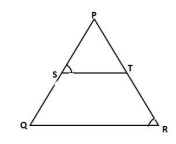
Since PS/SQ = PT/TR ∴ ST || QR (by converse of BPT)

∠PST = ∠PQR (Corresponding angles)

But ∠PST = ∠PRQ (given)

∠PQR = ∠PRQ

PR = PQ (sides opposite to equal angles are equal)

Hence ∆𝑃𝑄𝑅 is isosceles.

34. A medicine capsule is in the shape of a cylinder with two hemispheres stuck at each of its ends. The length of the entire capsule is 14mm and the diameter of the capsule is 5mm. Find its surface area.

View Answer

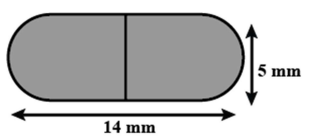
Ans. Diameter of cylinder and hemisphere = 5mm radius, (r) = 5/2

Total length = 14mm

Height of cylinder = 14 – 5 = 9mm

CSA of cylinder = 2⊼rh

= 2 x 22/7 x 5/2 x 9

= 990/7 mm²

CSA of hemispheres = 2⊼r ²

= 2x 22/7 x (5/2)²

= 275/7 mm²

CSA of 2 hemispheres = 2 x 275/7

= 550/7 mm²

Total area of capsule = 990/7 + 550/7

= 1540/7

= 220 mm²

A gulab jamun, contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 gulab jamuns, each shaped like cylinder with two hemispherical ends with length 5cm and diameter 2.8cm.

View Answer

Ans. Diameter of cylinder = 2.8 cm

𝑟𝑎𝑑𝑖𝑢𝑠 of cylinder = 2.8/2 = 1.4 cm

𝑟𝑎𝑑𝑖𝑢𝑠 of cylinder = 𝑟𝑎𝑑𝑖𝑢𝑠 of hemisphere = 1.4 cm

Height of cylinder = 5-2.8

= 2.2 cm

Volume of 1 Gulab jamun = vol. of cylinder + 2 x vol. of hemisphere

= ⊼ 𝑟 ²h + 2 x 2/3 ⊼ 𝑟 ³

22/7 x (1.4)² x 2.2 + 2 x 2/3 x 22/7 x (1.4)³

= 13.55 + 11.50

= 25.05 𝑐𝑚³

𝑣𝑜𝑙𝑢𝑚𝑒 𝑜𝑓 45 𝐺𝑢𝑙𝑎𝑏 𝑗𝑎𝑚𝑢𝑛 = 45 x25.05

𝑠𝑦𝑟𝑢𝑝 𝑖𝑛 45 𝐺𝑢𝑙𝑎𝑏 𝑗𝑎𝑚𝑢𝑛 = 30% x 45 x 25.05

= 30/100 x 45 x 25.05

= 338.175 cm³

≈ 338 cm³

35. The following table gives the distribution of the life time of 400 neon lamps:

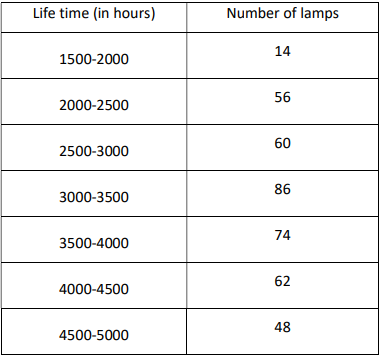
Find the average life time of a lamp

View Answer

Ans.

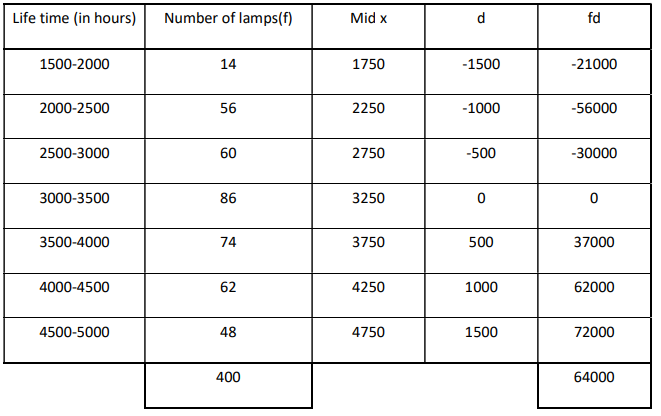
Mean = 3250 + 64000/400

= 3250 + 160

= 3410

Average life of lamp is 3410 hr.

SECTION E

36. Case Study 1

India is competitive manufacturing location due to the low cost of manpower and strong technical and engineering capabilities contributing to higher quality production runs. The production of TV sets in a factory increases uniformly by a fixed number every year. It produced 16000 sets in 6th year and 22600 in 9th year.

1) In which year, the production is 29,200 sets?

View Answer

Ans. a₆ =16000 a₉ = 22600

a + 5d = 16000——-(1)

a + 8d = 22600 ——–(2)

substitute a = 1600 – 5d from (1)

16000 – 5d + 8d = 22600

3d = 22600-16000

3d=6600

d = 6600/3

= 2200

a = 16000-5(2200)

a = 16000-11000

a = 5000

(i) a_n = 29200, a = 5000, d = 2200

a_n = a + (n-1) d

29200 = 5000 + (n – 1)2200

29200 – 5000 = 2200n – 2200

24200 + 2200 = 2200n

26400 = 2200n

n=264/12

n=12

in 12th year the production was Rs 29200

2) Find the production in the 8th year.

View Answer

Ans. n=8, a=5000, d=2200

a_n = a + (n-1)d

= 5000+(8-1)2200

= 5000+7 x 2200

= 5000+15400

= 20400

The production during 8th year is = 20400

Find the production in first 3 years.

View Answer

Ans. n = 3, a = 5000, d = 2200

s_n = n/2 [ 2a + (n-1)d]

= 3/2 [2(5000) + (3-1) 2200]

S₃ = 3/2 (10000 + 2 x 2200)

= 3/2 (10000 + 4400)

= 3 x 7200

= 21600

The production during first 3 year is 21600

3) Find the difference of the production in 7th year and 4th year.

View Answer

Ans. a₄ = a + 3d

= 5000 + 3 (2200)

= 5000 + 6600

= 11600

a₇ = a + 6d = 5000 + 6 x 2200

=5000 + 13200

= 18200

a₇ – a₄ = 18200-11600 = 6600

37. Case Study 2

Alia and Shagun are friends living on the same street in Patel Nagar. Shagun’s house is at the intersection of one street with another street on which there is a library. They both study in the same school and that is not far from Shagun’s house. Suppose the school is situated at the point O, i.e., the origin, Alia’s house is at A. Shagun’s house is at B and library is at C. Based on the above information, answer the following questions.

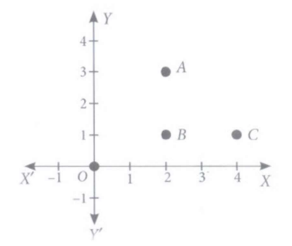
coordinates of A (2, 3) Alia’s house

coordinates of B (2, 1) Shagun’s house

coordinates of C (4,1) Library

(i) How far is Alia’s house from Shagun’s house?

View Answer

Ans. AB = √ (x₂ – x₁)² (y₂ – y₁)²

=√ (2 – 2)² (1 – 3)²

√0 + 4 = √4 = 2 units

𝐴𝑙𝑖𝑎′𝑠 ℎ𝑜𝑢𝑠𝑒 𝑓𝑟𝑜𝑚 𝑠ℎ𝑎𝑔𝑢𝑛′𝑠 ℎ𝑜𝑢𝑠𝑒 𝑖𝑠 2 𝑢𝑛𝑖𝑡

(ii) How far is the library from Shagun’s house?

View Answer

Ans. C (4,1), B (2,1)

CB = √(2 − 4)²+ (1 − 1)²

= √(−2)² + 0² = √4 + 0 = √4 = 2 unit

(iii) Show that for Shagun, school is farther compared to Alia’s house and library.

View Answer

Ans. 0(0,0), B(2,1)

OB = √ (2 − 0)² + (1 − 0)² = √2 ² + 1² = √4 + 1 = √5 units

Distance between Alia’s house and Shagun’s house, AB = 2 units

Distance between Library and Shagun’s house, CB = 2 units

OB is greater than AB and CB,

For shagun, school [O] is farther than Alia’s house [A] and Library [C]

Show that Alia’s house, shagun’s house and library for an isosceles right triangle.

View Answer

Ans. C (4, 1), A(2, 3) CA

= √ (2 − 4)² + (3 − 1)²

= √ (−2)² + 2²+

= √4 + 4 = √8

= 2√2 units AC²= 8

Distance between Alia’s house and Shagun’s house, AB = 2 units

Distance between Library and Shagun’s house, CB = 2 units

AB² + BC² = 2 ² + 2 ² = 4 + 4 = 8 = AC²

Therefore A, B and C form an isosceles right triangle.

38. Case Study 3

A boy is standing on the top of light house. He observed that boat P and boat Q are approaching the light house from opposite directions. He finds that angle of depression of boat P is 45° and angle of depression of boat Q is 30°. He also knows that height of the light house is 100 m.

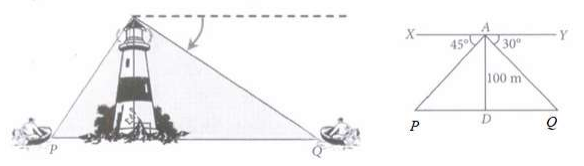
Based on the above information, answer the following questions.

(i) What is the measure of ∠APD?

View Answer

Ans. XY ║PQ and AP is transversal.

∠APD = ∠PAX (alternative interior angles)

∠APD=45˚

(ii) If ∠YAQ = 30°, then ∠AQD is also 30°, Why?

View Answer

Ans. Since XY || PQ and AQ is a transversal

so alternate interior angles are equal

hence ∠YAQ = ∠AQD=30˚

(iii) Find length of PD

View Answer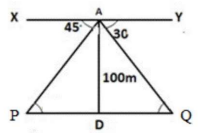

Ans. In ∆ 𝐴𝐷𝑃, θ = 45˚

tan 𝜃 = P/B

tan 45˚ = 100/PD

PD=100 m

Boat P is 100 m from the light house

Find length of DQ

View Answer

Ans. In ∆𝐴𝐷𝑄, θ = 30˚

tan 𝜃 = P/B

tan 30 = 100/DQ

= 1/√3 = 100/DQ

DQ = 100√3 m

Class X – Maths Basic Sample Paper – 1