Kindly share you feedback about the website – Click here
Mathematics
Class – IX
Time Allowed: 3 hours Maximum Marks: 80
General Instructions:
1. This question paper contains two parts A and B
2. Both Part A and Part B have internal choices
Part – A:
1. It consists of two section I and II
2. Section I has 22 questions. Internal choices provided in 7 questions.
3. Section II has Q.23 and 24 two case study-based questions. Each case study has 5 case –based sub parts. An examinee is to attempt any 4 out of 5 sub-parts.
Part – B:
1. Question No 25 to 31 are Very Short Answer type questions of 2 marks each
2. Question No 32 to 38 are Short Answer type questions of 3 marks each
3. Question No 39 to 41 are Long Answer type questions of 5 marks each.
4. Internal choice is provided in 2 questions of 2 marks, 2 questions of 3 marks and 1 question of 5 marks.
Part – A
Section – I
1. Write a rational number between √2 and √3
View AnswerAns. We know that,
√2 = 1.4142135….. and √3 = 1.732050807…
We see that 1.5 is a rational number which lies between 1.4142135….. and 1.732050807…
OR
How many rational numbers are there between 13 and 17?
View AnswerAns. There are infinite rational number between 13 and 17.
Eg. 13.4, 13.5……., 14.5, 14.6…….
2. Which of the following is irrational?
√4/7, √7
View AnswerAns. √4/7 = 2/7 is a rational number
√7 is an irrational number
3. Which one of the following is a polynomial?
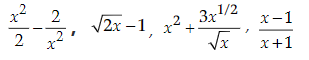
Ans.
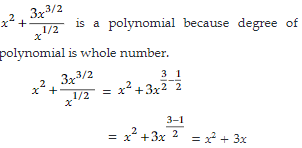
OR
How many zeroes are there in cubic equation?
View AnswerAns. 3 zeroes are there in a cubic equation
4. If (2, 0) is a solution of the linear equation 2x + 4y = k, then find the value of k
View AnswerAns. Put x = 2, y = 0 in the equation
2x + 3y = k
2(2) + 3(0) = k
4 + 0 = k
Hence, k = 4
5. What is the ordinate of the points that lie on the x-axis?
View AnswerAns. Because ordinate or y-coordinate of a point is perpendicular distance of this point from the x-axis measured along the y-axis. If a point lies on x-axis, then the perpendicular distance of a point from x-axis will be zero, so ordinate will be zero.
OR
In which quadrant, point (-3, -4) lies?
View AnswerAns. 3rd quadrant
6. The angles of a triangle are in the ratio 5: 3: 7. What is the type of the triangle?
View AnswerAns. Let the angles of triangle be 5x, 3x, 7x
According to question
5x + 3x + 7x = 180 (angle sum property)
15x = 180
x = 12
1st angle = 5 x 12 = 60
2nd angle = 3 x 12 = 36
3rd angle = 7 x 12 = 84
All the angles of the triangle are acute
The triangle is an acute angled triangle
7. If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is ___________ whose opposite angles are ___________.
View AnswerAns. PQRS is a quadrilateral whose opposite angles are supplementary.
8. ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140°, then find ∠BAC.
View AnswerAns. Given that,
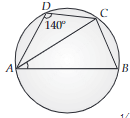
∠ADC + ∠ABC = 180
140 + ∠ABC = 180
∠ABC = 180 – 140
= 40
ABCD is a cyclic quadrilateral such that AB is the diameter of the circle circumscribing it.
Now, join AC. ∠C = 90 (Angles in a semi-circle is a right angle)
In ΔABC, we have
∠BAC = 180 – (90 + 40) = 50
9. The radius of a hemispherical balloon increases from 6 cm to 12 cm as air is being pumped into it. What is the ratios of the surface areas of the balloon in the two cases?
View AnswerAns. Radius r = 6 cm
Surface area = 3 πr2 = 3 π x 62 = 3 π x 6 x 6
Radius R = 12 cm
Surface area = 3 πR2 = 3 π x 122 = 3 π x 12 x 12
Ratio = 3 π x 6 x 6 : 3 π x 12 x 12
= 1 : 4
10. Factorize: x2 – 3x
View AnswerAns. x(x – 3)
11. ∠A + ∠B + ∠C is in ΔABC = __________
View AnswerAns. Sum of measures of all the angles of a triangle is 180
OR
A ray has __________ end point.
View AnswerAns. A ray has one end point
It is also called fixed point
12. What are the three steps from solids to points?
View AnswerAns. The three steps are: solids – surface – lines – points
13. Bisector of an angle divides it in _________ equal parts.
View AnswerAns. Two
14. The angles of a quadrilateral are in the ratio 2: 3: 6: 7. The largest angle of the quadrilateral is ________
View AnswerAns. Let the angle of the quadrilateral be 2x, 3x, 6x and 7x
2x + 3x + 6x + 7x = 360° (Angle sum property of quadrilateral)
Or 18x = 360°
X = 20°
Largest angle = 7x = 7 x 20° = 140°
15. Write an example of a constant polynomial
View AnswerAns. Constant polynomial is 7
OR
Name of the polynomial on the basis of degree: -3x + 2
View AnswerAns. Linear polynomial
16. In the given figure, PQ|| RS and EF || QS. If ∠PQS = 60°, then what will be the measure of ∠RFE?
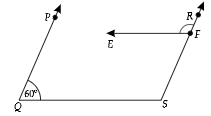
Ans. ∠PQS + ∠QSF = 180 (Consecutive Interior angle)
Or ∠PQS + ∠RFE = 180, as ∠QSF = ∠EFR
Or 60 + ∠RFE = 180 (corresponding angles)
∠RFE = 120
17. During maths lab activity, teacher give four sticks of length 6cm, 6 cm, 4 cm and 4 cm to each student to make different types of quadrilateral. Write the name of quadrilateral that can be formed with these sticks.

Ans. Kite, rectangle, parallelogram
Opposite sides of a parallelogram and a rectangle are equal. Also, adjacent sides of kite are equal. Thus kite, rectangle and parallelogram can be formed with the sticks of lengths 6 cm, 6 cm , 4 cm and 4 cm
18. Is trapezium is a parallelogram?
View AnswerAns. A trapezium has only one pair of parallel sides. Thus, it cannot be a parallelogram
19. Find any point on the line y = x
View AnswerAns. Every point on the line y = x has same value of x and y-coordinates, i.e., x = a and y = a. Hence (a, a) is a point on the line y = x
OR
In 5x – 8y = 17, express y in terms of x
View AnswerAns. 5x – 8y = 17
5x – 17 = 8y
y = (5/8)x – (17/8)
20. What is a straight line?
View AnswerAns. Two planes intersect each other to form a straight line
OR
Who was the teacher of Pythagoras?
View AnswerAns. Thales was the teacher of Pythagoras.
21. Can the angles 110, 80, 70 and 95 be the angles of a quadrilateral? Why or why not?
View AnswerAns. Here, sum of angles = 110 + 80 + 70 + 95 = 355
But we, know that the sum of angles of a quadrilateral is always 360°. Hence 110°, 80°, 70° and 95° cannot be the angles of a quadrilateral.
22. If x51 + 51 is divided by x + 1, then find remainder.
View AnswerAns. Let p(x) = x51 – 51 ……… (i)
i.e.,
p(-1) = (-1)51 + 51
= -1 + 51
= 50
Hence, remainder is 50
Section – II
23. Nikita has to make her project on ‘Monument in India’. She decided to make her project on Gol Gumbaz monument. She already known following things about it:

- It is located in a small town in Northern Karnataka
- It reaches up to 51 metres in height while the giant dome has an external diameter of 44 meters, making it one of the largest domes ever built.
- At each of the four corners of the cube is a dome shaped octagonal tower seven stories high with a staircase inside.
Help her in making project by answering the following questions:
A) What is the total area of a hemispherical dome?
i) 1/3 πr2
ii) 3 πr2
iii) 1/2 πr2
iv) 2 πr2
View AnswerAns. ii) 3 πr2
B) What is the curved surface area of hemispherical dome?
i) 908 πm2
ii) 968 πm2
iii) 340 πm2
iv) 780 πm2
View AnswerAns. ii) 968 πm2
Diameter = 44 m
Radius = 22 m
Curved surface area of hemispherical dome
= 2πr2
= 2π (22)2
= 968π m2
C) What is the volume of the sphere?
i) 4/3 πr3
ii) 2/3 πr3
iii) 1/3 πr3
iv) 1/2 πr3
View AnswerAns. i) 4/3 πr3
D) What is the circumference of the base of the dome?
i) 34 π
ii) 22 π
iii) 44 π
iv) 55 π
View AnswerAns. iii) 44 π
Circumference of the base of the dome = 2πr
= 2π(22)
= 44 π
E) Find the cost of painting the dome, given the cost of painting is Rs 100 per cm2
i) ₹980π
ii) ₹9690π
iii) ₹968000π
iv) ₹9700π
View AnswerAns. iii) ₹968000π
Curved surface area of hemispherical dome
= 968 π m2
Cost of painting 100 cm2 = ₹10
Cost of painting 1 m2 = ₹1000
Thus, cost of painting the dome = ₹1000 x 968 π cm2
= ₹968000 π
24. Prime Minister’s National Relief Fund (also called PMNRF in short) is the fund raised to provide support for people affected by natural and man-made disasters. Natural disasters that are covered under this include flood, cyclone, earthquake etc. Man-made disasters that are included are major accidents, acid attacks, riots, etc.

Two friends Sita and Gita, together contributed Rs 200 towards Prime Minister’s Relief Fund. Answer the following:
A) Which out of the following is not the equation in two variables?
i) 2x = 3
ii) 4 = 5x – 4y
iii) x2 + x = 1
iv) x – √2y = 3
View AnswerAns. iii) x2 + x = 1
x2 + x = 1 is not linear as highest power of 2. Also it is an equation in one variable. Thus, it is not a linear equation in two variables.
B) How to represent the above situation in linear equations in two variables?
i) 2x + y = 200
ii) x + y = 200
iii) 200x = y
iv) 200 + x = y
View AnswerAns. ii) x + y = 200
Here, x represents Sita’s contribution and y repreents Gita’s contribution.
C) If Sita contributed ₹76, then how much was contributed by Gita?
i) ₹120
ii) ₹123
iii) ₹124
iv) ₹125
View AnswerAns. iii) ₹124
If x = 76 then 76 + y = 200
y = 200 – 76
y = 124
D) If both contributed equally, then how much is contributed by each?
i) ₹50, ₹150
ii) ₹100, ₹100
iii) ₹50, ₹50
iv) ₹120, ₹120
View AnswerAns. ii) ₹100, ₹100
If x = y then x + x = 200
2x = 200
X = 100
Thus each contribution is ₹100
E) Which is the standard form of linear equations x = – 5?
i) x + 5 = 0
ii) 1.x – 5 = 0
iii) 1.x + 0.y + 5 = 0
iv) 1.x + 0.y = 5
View AnswerAns. iii) 1.x + 0.y + 5 = 0
Since, x= -5
- X + 5 = 0
Thus, standard form of x = -5 is 1.x + 0.y + 5 = 0
Part – B
25. If 7x = 1, then find the decimal expansion of x
View AnswerAns.

26. If (3x – 15°) and (x + 5°) are complementary angles, find the angles
View AnswerAns. (3x – 15°) + (x + 5°) = 90
4x = 90 + 10 = 100
X = 100/4 = 25
Angles are 60 and 30
OR
In the given figure, find the angles a and b and then show that LM || XY
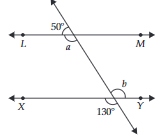
Ans. ∠a = 180 – 50
= 130 (Linear pair)
∠b = 130 (Since vertically opposite angles are equal)
This shows that ∠a = ∠b
But they are alternate interior angles.
LM || XY
27. In the figure, ΔABC and ΔDBC are two isosceles triangles on the same base BC. Prove that ∠ABD = ∠ACD
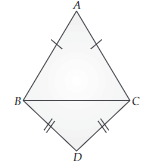
Ans. Join AD
In ΔABD and ΔACD,
AB = AC (Given)
BD = CD (Given)
AD = AD (Common)
By using SSS Congruency Rule
ΔABD ≅ ΔACD
∠ABD = ∠ACD (CPCT)
28. In the given figure, find the value of x.
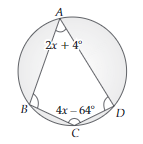
Ans. In a cyclic quadrilateral,
∠A + ∠C = 180 (opposite angles of cyclic quadrilateral are supplementary)
2x + 4 + 4x – 64 = 180
6x – 60 = 180
6x = 180 + 60
x = 240/6
x = 40
29. In the given figure, BC is a diameter of the circle and ∠BAO = 60°, then find ∠ADC
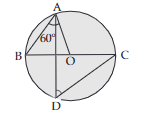
Ans. In ΔOAB,
OA – OB (Radii of the same circle)
∠ABO = ∠BAO (Angles opposite to equal sides are equal)
∠ABO = ∠BAO= 60 (Angles in the same segment of a circle are equal)
Therefore ∠ADC = 60
OR
In the figure, if ∠DAB = 65°, ∠ABD = 45°, then find ∠ACB.
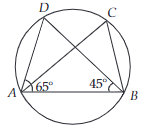
Ans. In ΔADB, using angle sum property
∠ABD + ∠ADB + ∠BAD = 180
45 + ∠ADB + 65 = 180
∠ADB = 180 – (45 + 65)
= 70
Thus, ACD = AOB = 70 (Angles in the same segment of a circle are equal)
30. Find the area of a triangle, two sides of which are 8 cm and 11 cm and the perimeter is 32 cm.
View AnswerAns. Let the side AB(c) = 8 cm
Side AC(b) = 11 cm
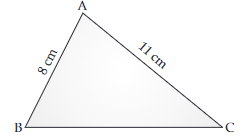
Perimeter of ΔABC = 32 cm
a + b + c = 32 cm
a + 8 + 11 = 32
a = 32 – 19 = 13 cm
ar(ΔABC) = |√s(s – a)(s – b)(s – c)|
ar(ΔABC) = |√16(16 – 13)(16 – 8)(16 – 11)|
= |√16 x 3 x 8 x 5|
= |√4 x 4 x 2 x 2 x 2 x 3 x 5|
= |4 x 2√2 x 3 x 5|
ar(ΔABC) = 8√30 cm2
31. Coronavirus disease (COVID-19) is an infectious disease. It is caused by a newly discovered coronavirus. Most people who fall sick with COVID-19 experience mild to moderate symptoms and recover without special treatment
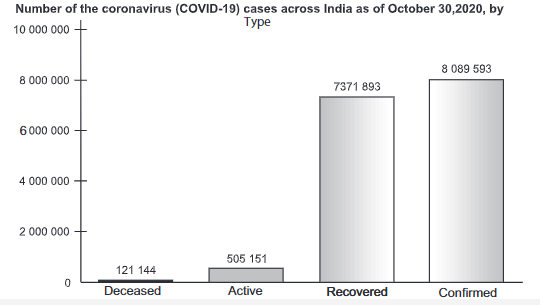
Coronavirus is mainly transmitted through droplets generated when an infected person coughs, sneezes or exhales. Look at the bar graph and answer the following:
i) What is horizontal line representing?
View AnswerAns. Number of cases
ii) How many confirmed cases were more than recovered cases?
View AnswerAns. Required number = confirmed cases – recovered cases
= 8089593 – 7371898
= 717695
32. Find three rational numbers between 5/7 and 9/11.
View AnswerAns.

OR
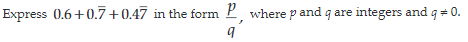
Ans.
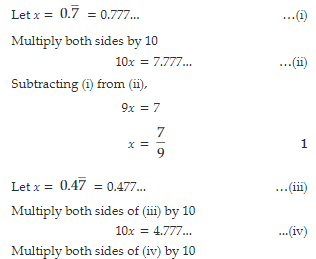
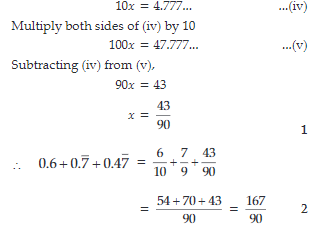
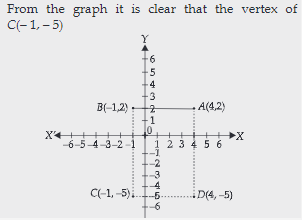
33. Point A(4, 2), B(-1, 2) and D(4, -5) are three vertices of a rectangle ABCD. Plot these points and hence find the vertex C.
View AnswerAns.
34. If f(x) = x3 – 3x2 + 3x – 4, find f(2) + f(-2) + f(0)
View AnswerAns. Given f(x) = x3 – 3x2 + 3x – 4
f(2)= (2)3 – 3(2)2 + 3(2) – 4
= 8 – 12 + 6 – 4
f(2) = -2
and f(-2) = (-2)3 – 3(-2)2 + 3(-2) – 4
= -8 – 12 – 6 – 4
f(-2) = -30
And f(0) = -4
Therefore f(2) + f(-2) +f(0) = -2 – 30 – 4 = -36
OR
Draw the quadrilateral ABCD whose vertices are A(0, 0), B(5, 0), C(3, 2) and D(0, 2)
View AnswerAns.
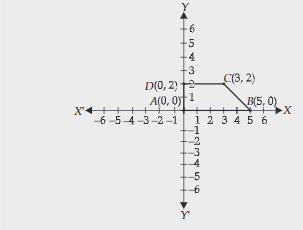
35. Prove that if two lines intersect, vertically opposite angles are equal.
View AnswerAns. Given Two lines AB and CD intersect at O
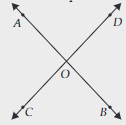
To prove
i) ∠AOC = ∠BOD
ii) ∠AOD = ∠BOC
Proof: Ray OA stands on line CD
Therefore ∠AOC + ∠AOD = 180 (linear pair) ——(i)
Again ray OD stands on line AB
Therefore ∠AOD + ∠BOD = 180 (linear pair) ——(ii)
From eqn (i) and (ii)
∠AOC + ∠AOD = ∠AOD + ∠BOD (Each equal to 180)
Therefore ∠AOC = ∠BOD
Similarly ∠AOD = ∠BOC
36. Find the area of a triangular field of sides 18 m, 24 m and 30 m. Also find the altitude corresponding to the shortest side.
View AnswerAns. s = (18 + 24 + 30)/2 = 36 m
Area = √s(s – a)(s – b)(s –c)
= √36(36 – 18)(36 – 24)(36 – 30)
= √36 x 18 x 12 x 6
= 216 m2
Altitude (height) corresponding to the shortest side
= 2 x Area/Base = 2 x 216/18 = 24 m
37. How many litres of milk can a hemispherical bowl of diameter 10.5 cm hold? Use π = 3.14)
View AnswerAns. Given, diameter of hemispherical bowl = 10.5 cm
Radius of hemispherical bowl r = 10.5/2 = 5.25 cm
Volume of hemispherical bowl = 2/3 πr3
= 2/3 x 3.14 x (5.25)3
= 302.91
= 303 cm3
Therefore, Amount of milk that the hemispherical bowl can hold = 0.303 litres
38. ABCD is a rhombus. Show that the diagonal AC bisect ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.
View AnswerAns. Given ABCD is a rhombus
To prove:
∠BAC = ∠DAC and ∠DCA = ∠BCA
So, AB = BC = CD = AD
In ΔADC and ΔABC
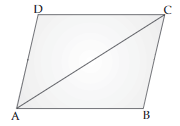
AB = AD (given)
CD = CB (given)
AC = AC (common)
So, ΔADC ≅ ΔABC (By SSS Congruency Rule)
So, ∠DAC = ∠BAC (CPCT)
Similarly,
ΔDCA ≅ ΔBCA
∠DCA = ∠BCA (CPCT) Hence proved
39 a) The blood groups of 30 students of Class IX are recorded as follows:
A, B, O, O, AB, O, A, O, B, A, O, B, A. O, O
A, AB, O, A, A, O, O, AB, B, A, O, B, A, B, O
i) Represent this data in the form of a frequency distribution table
ii) Which is the most common and which the rarest blood group among these students?
View AnswerAns. i) Frequency Distribution Table:
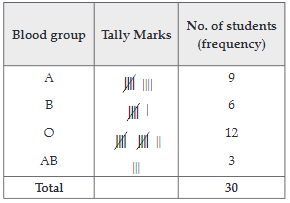
ii) Blood group ‘O’ is most common as it has highest frequency i.e., 12. Blood group AB is rarest.
b) Facts or information collected with a definite purpose are called.
View AnswerAns. Data
40. In the given figure, ABCD and BPQ are straight lines. If BP = BC and DQ is parallel to CP prove that:
i) CP = CD
ii) DP bisects CDQ
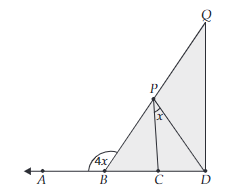
Ans. BP = BC (Given)
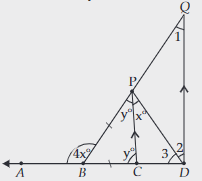
∠BCP = ∠BPC
= y
∠1 = y (corresponding angles)
∠2 = x (Alternate angles)
∠3 = ∠1 + ∠2 (external angle)
= x + y
4x = 2y (external angle)
∠2 = x (Alternate angles)
Y = x + ∠3
Or x = ∠3
Or CP = CD
Also, we have ∠2 = x, x = ∠3
Or DP bisects ∠CDQ
Hence proved
OR
A student Amit of class IX is unable to write in his examination, due to fracture in his arm. Akhil a student of class VI writes for him. The sum of their ages is 25 years.
i) Write a linear equation for the above situation and represent it graphically.
ii) Find the age of Akhil from the graph, when age of Amit is 14 years
View AnswerAns. Let age of Amit = x years
Age of Akhil = y years
i) According to the question the linear equation for the above situation is
x + y = 25
or y = 25 – x
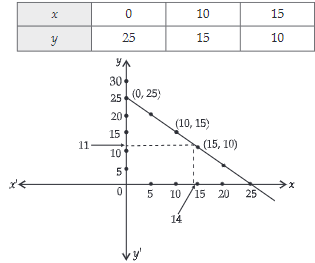
ii) From the graph when Amit’s age = 14 years, then Akhil’s age = 11 years
41. It is known that if a + b = 10, then a + b – c = 10 – c. Write the Euclid’s axiom that best illustrates this statement. Also give two more axioms other than the axiom used in the above situation.
View AnswerAns. Axiom: If equals are subtracted from equals, the remainders are equal.
Two more axioms:
i) Things which are halves of the same thing are equal to one other
ii) The whole is greater than the part